Zero-skipping average

In some cases, in order to avoid possible singularities, values $C_j=0$ are skipped. Then if $N_{\mathrm{obs}}^*$ is the number of non-zero data points, we can evaluate the 'zero-skipping' average as

$$x=\langle x\rangle^*={\ensuremath{\displaystyle{\frac{{\ensuremat... ...obs}}}}}}{{\ensuremath{\displaystyle{N_{\mathrm{obs}}^*}}}}}}}\langle x\rangle$$

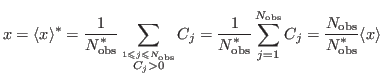
The standard deviation is then

$$\sigma_{x^*}= {\ensuremath{\displaystyle{\frac{{\ensuremath{\disp... ...e{\langle x\rangle^*}}}}{{\ensuremath{\displaystyle{N_{\mathrm{obs}}^*}}}}}}}}$$

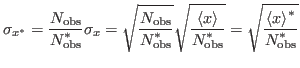
Note that the s.d. is evaluated exactly as if the non-zero $C_j$ were the only observations, whilst the average is overestimated by the fraction of zero-counting events.