Simple average

Suppose we have $N_{\mathrm{obs}}$ Poisson-variate experimental evaluations $C_j,\quad j=1\ldots N_{\mathrm{obs}}$ , of the same quantity $x$ . There are different ways to obtain from all $N_{\mathrm{obs}}$ data values a single estimate of the observable which is better than any of them. The most straightforward and the best is the simple average

$$x=\langle x\rangle={\ensuremath{\displaystyle{\frac{{\ensuremath{... ...aystyle{ N_{\mathrm{obs}}}}}}}}} \mathop{\sum}_{j=1}^{N_{\mathrm{obs}}}C_j\ .$$

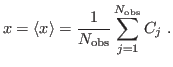
As the sum of Poisson variates is a Poisson variate, the standard deviation

$$\sigma_x=\sqrt{\langle x^2\rangle-\langle x\rangle^2}=\sqrt{ {\en... ...\mathrm{obs}}}}}}}}} \mathop{\sum}_{j=1}^{N_{\mathrm{obs}}}C_j }\right)}} }$$

can be evaluated more comfortably as

$$\sigma_x={\ensuremath{\displaystyle{\frac{{\ensuremath{\displayst... ...style{\langle x\rangle}}}}{{\ensuremath{\displaystyle{N_{\mathrm{obs}}}}}}}}}}$$